Disclaimer of Liability
The William Margrabe Group, Inc. makes no guarantee or warranty of any kind--including express or implied--concerning the relevance, accuracy, reliability, suitability for any purpose, etc. of the content at this site ("Reward-andRisk-Analytics . com") or at other sites to which it links. We provide all content, “as is”. (click here to read the rest of the disclaimer.)
Terms of Service
If you gain access to any information on this website, you are bound by the terms and conditions in the terms of service ("TOS") agreement (click here) and the Privacy Agreement (click here). If you don't want that, you must leave this web site, immediately.
Video and Slideshow
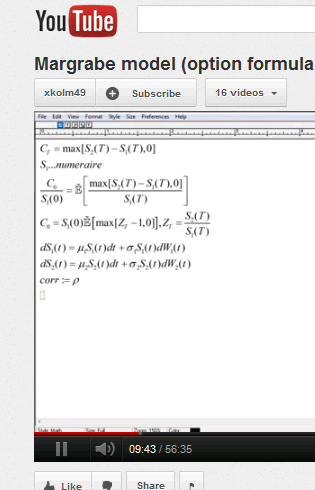
"Margrabe model (option formula). Change of numeraire" (YouTube, 5/12/12)
The "un-authorized", long-awaited, full-length (almost: 56+ minutes!) "feature film" deriving the value of a Margrabe option is now available. Thanks, xcolm49!
"Monte Carlo for the Margrabe Option" (Bocconi Univ. via Slideshare, 1/21/12)
Available, now, as a slide show: Monte Carlo pricing for a Margrabe option. It, too, is "unautho-rized".
News Room
The 35th anniversary of the publication of Margrabe (1978) is approaching. Prepare to party like it's 1978.
Reference Room
William Margrabe, "The Value of an Option to Exchange One Asset for Another," Journal of Finance 33(1) (March 1978), pp. 177-186.
This article is available from these sources:
It is also chapter 12 in Options; Classic Approaches to Pricing and Modelling (Lane Hughston, ed.). London: Risk Books, 1999.
This book's sixteen chapters are all reprints of classic papers on option pricing, which Fischer Black and Myron Scholes, Robert Merton, Paul Samuelson, and other scholars published originally in scholarly journals. Any scholar or practitioner with a serious interest in options might consider putting this reference book in his library.
RewardandRiskAnalytics .com
RewardAndRiskAnalytics.com is our website about that subset of Analytics (the study and collection of information about methods of analysis) associated with RewardAndRisk . For example, Markowitz Portfolio analysis is just one form of analysis of RewardAndRisk.
The William Margrabe Group, Inc.
The William Margrabe Group, Inc. (WMG, Inc.; the Group), a consulting firm since 1994, provides clients with goods and services related to mathematical and statistical models for business purposes. Almost all of these business models have been economic models, of which almost all have been financial models, of which most have been models for pricing and managing the risk of derivative products (such as swaps and options), bonds, and money market instruments. The underlying risk factors have include prices of bonds, currencies, real estate, and shares; yields of money market instruments, notes, and bonds; degree-days, default risk; mortality risk; indexes of the preceding; spot, forward, and futures prices; functions of the preceding; etc.
William Margrabe
William Margrabe is the President of The William Margrabe Group, Inc. and its head of research. After graduating from The Johns Hopkins University with his B.A. (economics, Phi Beta Kappa), he earned his Ph.D. (economics, concentrations in finance and economic development) from the University of Chicago.
His first career after graduate school was teaching finance and real estate at Wharton, NYU, and other graduate schools of business. While at Chicago he began his pioneering research about the value of an option to exchange one asset for another, and he finished and published it, when he was at Wharton.
His second career was providing quantative support concerning all aspects of financial models (mainly, models of valuation and risk management) for financial product sales and trading operations at Freddie Mac, Bankers Trust, Morgan Stanley, and Salomon Brothers.
His third career has been providing consulting services for clients concerning the same models and matters that were in his domain, when he was working in New York.
The "Margrabe Option"
A "Margrabe option" is an option to exchange one asset for another. Other names for this option include "exchange option" and "swap option".
It's payoff function is
Payoff = Max(0, X1 - X2),
where X1 and X2 are the underlying asset values.
It is a "Rainbow option", so-called, because it can have a "spectrum" (in this case, with just two members) of underlying assets.
Many financial contracts have this sort of payoff function:
- investment performance incentive fee,
- cross-currency option contract,
- an "Asian" option on the average value of a price over time, with constant strike price,
- an "Asian" option to pay or receive the average price over time for a commodity or asset,
- the value of a pension plan sponsor's over-funding or under-funding of a (simplified) plan,
- a "spark spread" option, e.g., as used to value a gas-fired power plant (buy gas, sell power),
- et cetera.
The "Margrabe Model"
The "Margrabe Model" (Margrabe, 1978) is an option pricing model that gives the value for a Margrabe option, when the two underlying asset prices are log normal random variables, the variance of the logarithm of the ratio of the underlying price variables is constant (e.g., the correlation coefficient and the variances are constant), and other "Black-Scholes-Merton" assumptions hold.
William Margrabe's first consulting project in New York was Fischer Black arranged in the middle 1980s for Margrabe to fly to New York to help one of the talented C-programmers in Black's group at Goldman Sachs to implement the Margrabe model.